Fitxer:Damped spring.gif
Aparença
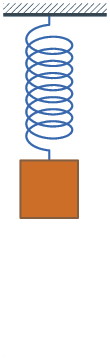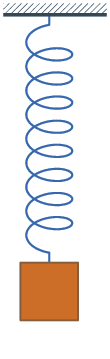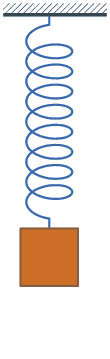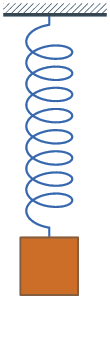
Damped_spring.gif (110 × 359 píxels, mida del fitxer: 207 Ko, tipus MIME: image/gif, en bucle, 65 fotogrames, 4,6 s)
Historial del fitxer
Cliqueu una data/hora per veure el fitxer tal com era aleshores.
| Data/hora | Miniatura | Dimensions | Usuari/a | Comentari | |
|---|---|---|---|---|---|
| actual | 18:11, 11 oct 2008 | 110 × 359 (207 Ko) | Nard the Bard | replace lost file | |
| 04:54, 24 juny 2007 | Sense miniatura | 110 × 359 (207 Ko) | Oleg Alexandrov | Illustration of en:Damping {{Information |Description= |Source=self-made with en:Matlab. Converted to gif animation with the en:ImageMagick convert tool (see the specific command later in the code). |Date= 02:42, 24 June 2007 (UTC) |Autho |
Ús del fitxer
La pàgina següent utilitza aquest fitxer:
Ús global del fitxer
Utilització d'aquest fitxer en altres wikis:
- Utilització a ar.wikipedia.org
- Utilització a arz.wikipedia.org
- Utilització a cs.wikipedia.org
- Utilització a cv.wikipedia.org
- Utilització a cy.wikipedia.org
- Utilització a da.wikipedia.org
- Utilització a de.wikipedia.org
- Utilització a de.wikibooks.org
- Utilització a en.wikipedia.org
- Utilització a en.wikibooks.org
- Utilització a et.wikipedia.org
- Utilització a fa.wikipedia.org
- Utilització a fi.wikipedia.org
- Utilització a fi.wiktionary.org
- Utilització a fr.wikipedia.org
- Utilització a fr.wikibooks.org
- Utilització a ga.wikipedia.org
- Utilització a he.wikipedia.org
- Utilització a hi.wikipedia.org
- Utilització a hy.wikipedia.org
- Utilització a it.wikipedia.org
- Utilització a ja.wikipedia.org
- Utilització a ko.wikipedia.org
- Utilització a lv.wikipedia.org
- Utilització a mk.wikipedia.org
- Utilització a myv.wikipedia.org
- Utilització a nl.wikipedia.org
- Utilització a ru.wikipedia.org
- Utilització a simple.wikipedia.org
- Utilització a sk.wikipedia.org
- Utilització a sq.wikipedia.org
- Utilització a sv.wikipedia.org
- Utilització a uk.wikipedia.org
- Utilització a uz.wikipedia.org
- Utilització a vi.wikipedia.org
- Utilització a www.wikidata.org
- Utilització a zh.wikipedia.org