Usuari:EstherBave/proves

El valor absolut és la distància d'un nombre a l'origen de coordenades

En matemàtiques, donat un nombre real a s'anomena valor absolut d'a, i es denota per |a|, a la distància d'aquest nombre a l'origen de coordenades. En altres paraules, és el mateix nombre prescindint del signe algebraic (+ o -). Atès que es defineix com a una distància, el seu valor sempre és estrictament positiu llevat del zero, que te valor absolut zero.

Es pot generalitzar el concepte de valor absolut en una gran varietat de contextos: nombres complexos, quaternions, anells ordenats, i espais vectorials. Per a nombres complexos el valor absolut s'anomena mòdul del nombre i representa la distància del punt a l'origen. En espais vectorials de dimensió superior a 1, s'anomena norma del vector i representa la longitud del vector.

Definició and propietats

Per a tot nombre real a, el seu valor absolut es representa per |a| (el nombre entre dues barres verticals) i es defineix com

$|a|=\left\{{\begin{array}{rl}a,&{\text{if }}a\geq 0\\-a,&{\text{if }}a<0.\end{array}}\right.$

Des del punt de vista geomètric, el valor absolut representa la distància a l'origen del punt situat en la coordenada a sobre la recta dels nombres reals. La distància entre dos nombres reals a i b es pot expressar com el valor absolut de la diferència |a-b|.

Una definició equivalent s'obtè usant que l'arrel quadrada representa la única arrel positiva d'un nombre real positiu:

$|x|={\sqrt {x^{2}}}$

Aquesta definició és emprada per molts llenguatges de programació que contenen la funció ABS() definida com

$\operatorname {ABS} (x)=|x|=+{\sqrt {x^{2}}}$

El valor absolut té les propietats següents ($a$i $b$representen nombres reals):

1. ${\left|a\right|}\geq 0\,$
2. ${\left|a\right|}=0\Leftrightarrow a=0\,$
3. ${\left|a\cdot b\right|}=\left|a\right|\cdot \left|b\right|\,$
4. Desigualtat triangular: ${\left|a+b\right|}\leq {\left|a\right|+\left|b\right|}\,$

Altres propietats són (falta referencia de demostracions):

1. ${{\big |}\left|a\right|{\big |}=|a|}$
2. $|-a|=|a|$
3. $|a-b|=0\iff a=b$
4. $|a-b|\leq |a-c|+|c-b|$
5. $\left|{\frac {a}{b}}\right|={\frac {|a|}{|b|}}\$ (si $b\neq 0$)
6. $|a-b|\geq {\big |}\,|a|-|b|\,{\big |}$
7. $|a|\leq b\iff -b\leq a\leq b$
8. $|a|\geq b\iff a\leq -b\$ o $a\geq b$

Funció valor absolut

Valor absolut de la funció |x|

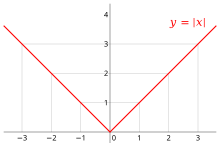
La funció $f(x)=|x|$valor absolut és contínua a tota la recat real. És monòtona decreixent a l'interval $(-\infty ,0]$i monòtona creixent a $[0,\infty )$

l absolute value function is continuous everywhere. It is differentiable everywhere except for x = 0. It is monotonically decreasing on the interval Plantilla:Open-closed and monotonically increasing on the interval Plantilla:Closed-open. Since a real number and its opposite have the same absolute value, it is an even function, and is hence not invertible. The real absolute value function is a piecewise linear, convex function.

Both the real and complex functions are idempotent.

Relationship to the sign function

The absolute value function of a real number returns its value irrespective of its sign, whereas the sign (or signum) function returns a number's sign irrespective of its value. The following equations show the relationship between these two functions:

$|x|=x\operatorname {sgn}(x),$

or

$|x|\operatorname {sgn}(x)=x,$

and for x ≠ 0,

$\operatorname {sgn}(x)={\frac {|x|}{x}}={\frac {x}{|x|}}.$

Derivative

The real absolute value function has a derivative for every x ≠ 0, but is not differentiable at x = 0. Its derivative for x ≠ 0 is given by the step function:

${\frac {d|x|}{dx}}={\frac {x}{|x|}}={\begin{cases}-1&x<0\\1&x>0.\end{cases}}$

The subdifferential of |x| at x = 0 is the interval Plantilla:Closed-closed.

The complex absolute value function is continuous everywhere but complex differentiable nowhere because it violates the Cauchy–Riemann equations.

The second derivative of |x| with respect to x is zero everywhere except zero, where it does not exist. As a generalised function, the second derivative may be taken as two times the Dirac delta function.

Antiderivative

The antiderivative (indefinite integral) of the real absolute value function is

$\int |x|dx={\frac {x|x|}{2}}+C,$

where C is an arbitrary constant of integration. This is not a complex antiderivative because complex antiderivatives can only exist for complex-differentiable (holomorphic) functions, which the complex absolute value function is not.

Generalitzacions

Espais Vectorials

Categoria:Nombres reals Categoria:Funcions